This project, through a transdisciplinary enquiry, explores the inherent, morphological oneness between natural dynamical systems and the process of musical composition. Both processes, the former being determined through laws of physics, and the latter through cogonition, follow generative, emergent principles that lead to the evolution of their form.
Can a musical composition be re-imagined as a generative cognitive territory that is a populated with notes?
The set of mass and momentum Navier-Stokes (NS)equations require an initial condition and a boundary condition to fully describe the flow conditions, so that the unknown quantity such as velocity field of the flow, in space and time, can be obtained from these equations, following the governing laws of natural physics.
In a very similar fashion, a musical field can be obtained from some initial conditions and boundary conditions, following the governing laws of ragas, for example in classical music parlance. Often, the evolving phenomenon gets enriched due to the fact that even if the governing laws of the ragas are strictly followed, the initial and boundary conditions of the temporally evolving musical performance is a cognitive function of the individual performer.
Fall 2019
Transient Territories: Re-imagining fluids as tectonic composers
Mentored by Professor Sameer Khandekar (also my father)
In collaboration with Indian Institute of Technology Kanpur, India
The differential equation of motion of fluid particles, which is a direct outcome of application of Newtons second law of motion to fluids, which deform under the action of applied stress/force. Simplified and deterministic solutions of NS equation are indeed available for simple low inertia fluid systems, or ‘laminar’ flows. With increasing inertia, the flow becomes progressively more indeterministic, making its journey from ‘laminar’, all the way transiting to highly ‘turbulent’ flows. For the latter case, suitable modifications in the NS-equations are needed to handle the stress-deformation relationship, which becomes increasingly complex. This is akin to bringing an order, within the apparent disorder of the highly turbulent nature of the flow.
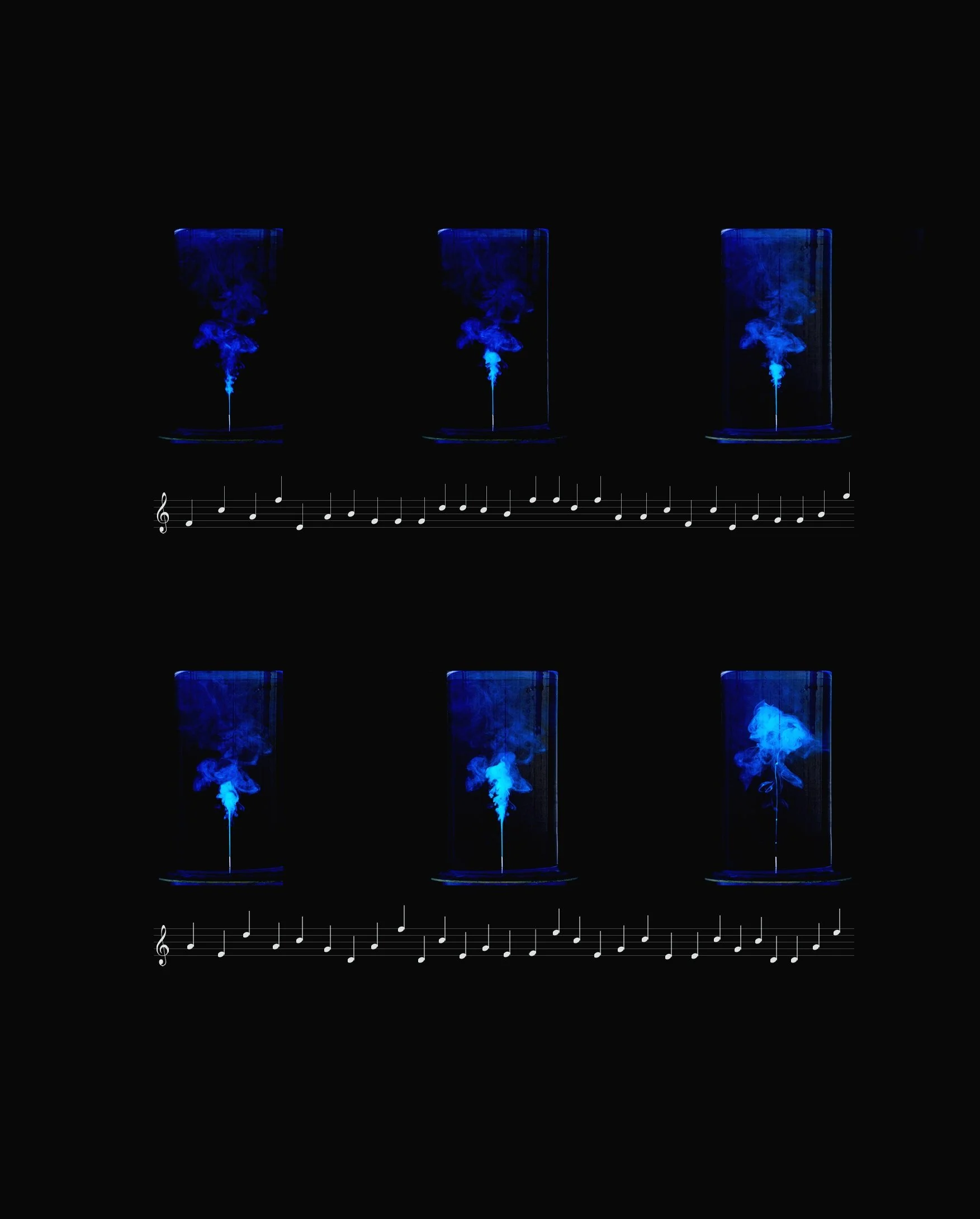
These two asymptotes, from laminar to turbulent can be morphologically mapped to deterministic musical notes on one hand (Laminar music) and pure white noise on the other (fully turbulent music).
Thus, the spectrum of the differential NS equation provides a very sound basis of imagining muasic, with the musical notes mapped to the local flow velocities (momentum) or concentration of particles (mass) in the flow.